Finding Characteristic Roots Example 2

Finding Characteristic Roots Example 2 Youtube The characteristic polynomial of a is the function f(λ) given by. f(λ) = det (a − λin). we will see below, theorem 5.2.2, that the characteristic polynomial is in fact a polynomial. finding the characterestic polynomial means computing the determinant of the matrix a − λin, whose entries contain the unknown λ. The characteristic polynomial of this recurrence is defined as the polynomial for example, let be the th fibonacci number defined by , and then, its characteristic polynomial is . the roots of the polynomial can be used to write a closed form for the recurrence. if the roots of this polynomial are distinct, then suppose the roots are .

Linear Second Order De Characteristic Equation With Real Distinct For example, \(a n = 2a {n 1} a {n 2} 3a {n 3}\) has characteristic polynomial \(x^3 2 x^2 x 3\text{.}\) assuming you see how to factor such a degree 3 (or more) polynomial you can easily find the characteristic roots and as such solve the recurrence relation (the solution would look like \(a n = ar 1^n br 2^n cr 3^n\) if there. We start with the differential equation. ay′′ by′ cy = 0 a y ″ b y ′ c y = 0. write down the characteristic equation. ar2 br c = 0 a r 2 b r c = 0. solve the characteristic equation for the two roots, r1 r 1 and r2 r 2. this gives the two solutions. y1(t) = er1t and y2(t) = er2t y 1 (t) = e r 1 t and y 2 (t) = e r 2 t. Example \(\pageindex{1}\) general solution; example \(\pageindex{2}\): graphical solutions; contributors and attributions; we have already addressed how to solve a second order linear homogeneous differential equation with constant coefficients where the roots of the characteristic equation are real and distinct. Characteristic polynomial definition. assume that a is an n×n matrix. hence, the characteristic polynomial of a is defined as function f (λ) and the characteristic polynomial formula is given by: f (λ) = det (a – λin) where i represents the identity matrix. the main purpose of finding the characteristic polynomial is to find the eigenvalues.

Find The Characteristic Equation Roots Of The Matrix Example Part 3 Example \(\pageindex{1}\) general solution; example \(\pageindex{2}\): graphical solutions; contributors and attributions; we have already addressed how to solve a second order linear homogeneous differential equation with constant coefficients where the roots of the characteristic equation are real and distinct. Characteristic polynomial definition. assume that a is an n×n matrix. hence, the characteristic polynomial of a is defined as function f (λ) and the characteristic polynomial formula is given by: f (λ) = det (a – λin) where i represents the identity matrix. the main purpose of finding the characteristic polynomial is to find the eigenvalues. A root because [1;1;1]t is an eigenvector as it is the sum of the row elements. so, we can divide ( 3 15 2 24 360)=(15 ) which is 24 x2. so, we have p a( ) = (15 )(i p 24 )( i p 24 ). problem: find the characteristic polynomial of the boolean matrix encoding one of the solutions of the 8 queen problems a= 2 6 6 6 6 6 6 6 6 6 4 1 0 0 0 0 0. We say that the eigenvalue 5 in this example has multiplicity 2, because \((\lambda 5)\) occures two times as a factor of the characteristic polynomial. in general, the mutiplicity of an eigenvalue \(\lambda\) is its multiplicity as a root of the characteristic equation. example.

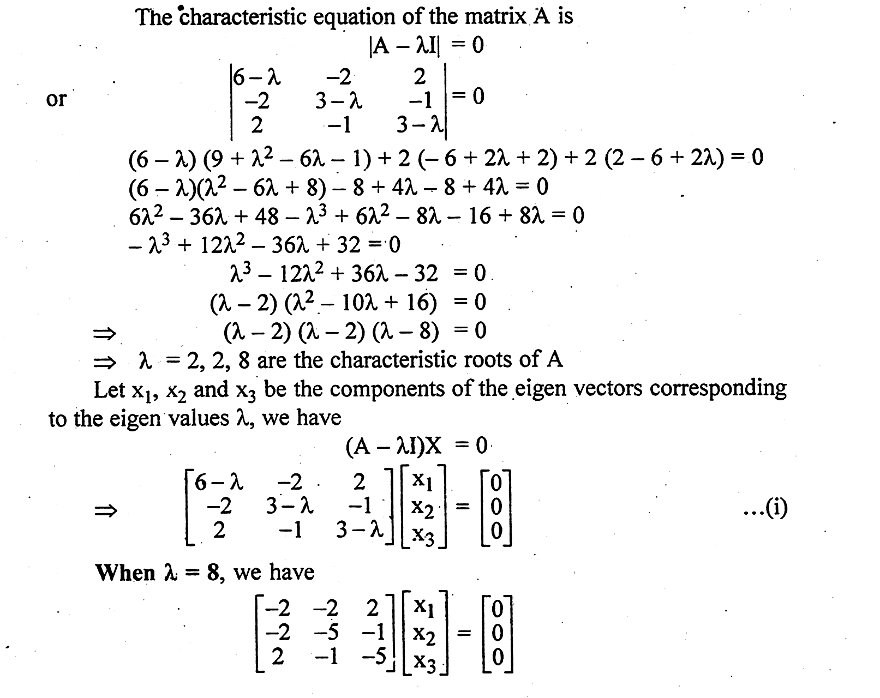
Find The Characteristic Roots Of Matrix A 6 2 2 2 3 1 2 1 A root because [1;1;1]t is an eigenvector as it is the sum of the row elements. so, we can divide ( 3 15 2 24 360)=(15 ) which is 24 x2. so, we have p a( ) = (15 )(i p 24 )( i p 24 ). problem: find the characteristic polynomial of the boolean matrix encoding one of the solutions of the 8 queen problems a= 2 6 6 6 6 6 6 6 6 6 4 1 0 0 0 0 0. We say that the eigenvalue 5 in this example has multiplicity 2, because \((\lambda 5)\) occures two times as a factor of the characteristic polynomial. in general, the mutiplicity of an eigenvalue \(\lambda\) is its multiplicity as a root of the characteristic equation. example.