Example Stokes Theorem 2

Example Stokes Theorem 2 Youtube Figure 16.7.1: stokes’ theorem relates the flux integral over the surface to a line integral around the boundary of the surface. note that the orientation of the curve is positive. suppose surface s is a flat region in the xy plane with upward orientation. then the unit normal vector is ⇀ k and surface integral. C c has a counter clockwise rotation if you are above the triangle and looking down towards the xy x y plane. see the figure below for a sketch of the curve. solution. here is a set of practice problems to accompany the stokes' theorem section of the surface integrals chapter of the notes for paul dawkins calculus iii course at lamar university.

Example Of Stokes Theorem Youtube Example 1. let c be the closed curve illustrated below. for f(x, y, z) = (y, z, x), compute ∫cf ⋅ ds using stokes' theorem. solution: since we are given a line integral and told to use stokes' theorem, we need to compute a surface integral ∬scurlf ⋅ ds, where s is a surface with boundary c. we have freedom to choose any surface s, as. Example. let’s put all of this new information, along with our previously learned skills, to work with an example. suppose f → = x 2, 2 x y x, z . let c be the circle x 2 y 2 = 1 in the plane z = 0 oriented counterclockwise, and let s be the disk x 2 y 2 ≤ 1 oriented with the normal vector k →. verify stoke’s theorem by. In this theorem note that the surface s s can actually be any surface so long as its boundary curve is given by c c. this is something that can be used to our advantage to simplify the surface integral on occasion. let’s take a look at a couple of examples. example 1 use stokes’ theorem to evaluate ∬ s curl →f ⋅ d →s ∬ s curl f. Stokes’ theorem. let s be a piecewise smooth oriented surface with a boundary that is a simple closed curve c with positive orientation (figure 6.79).if f is a vector field with component functions that have continuous partial derivatives on an open region containing s, then.
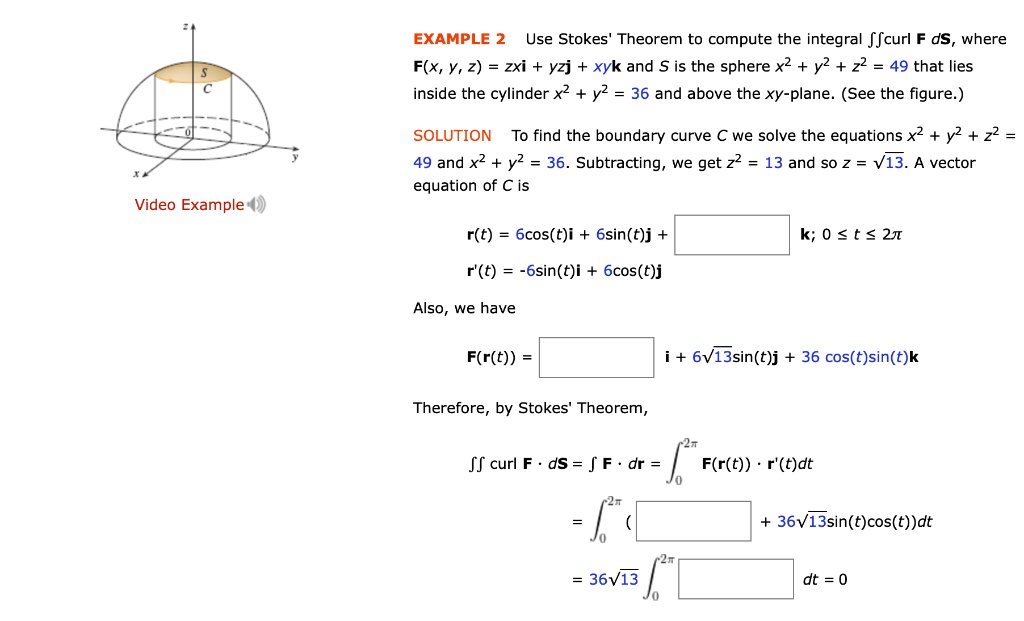
Solved Example 2 Use Stokes Theorem To Compute The Integral гўл в Curl In this theorem note that the surface s s can actually be any surface so long as its boundary curve is given by c c. this is something that can be used to our advantage to simplify the surface integral on occasion. let’s take a look at a couple of examples. example 1 use stokes’ theorem to evaluate ∬ s curl →f ⋅ d →s ∬ s curl f. Stokes’ theorem. let s be a piecewise smooth oriented surface with a boundary that is a simple closed curve c with positive orientation (figure 6.79).if f is a vector field with component functions that have continuous partial derivatives on an open region containing s, then. Stokes’ theorem relates a flux integral over a non complete surface to a line integral around its bound ary. example compute the flux integral rr s r fds where s is the part of the paraboloid z = x2 y2 inside the cylinder x2 y2 = 4 oriented upward, and f(x,y,z) = x2z2i y2z2j xyzk. rather than evaluating rr s curlf ds, we simply compute. Stokes' theorem says that ∮c ⇀ f ⋅ d ⇀ r = ∬s ⇀ ∇ × ⇀ f ⋅ ˆn ds for any (suitably oriented) surface whose boundary is c. so if s1 and s2 are two different (suitably oriented) surfaces having the same boundary curve c, then. ∬s1 ⇀ ∇ × ⇀ f ⋅ ˆn ds = ∬s2 ⇀ ∇ × ⇀ f ⋅ ˆn ds. for example, if c is the unit.

Stokes Theorem Example 2 Youtube Stokes’ theorem relates a flux integral over a non complete surface to a line integral around its bound ary. example compute the flux integral rr s r fds where s is the part of the paraboloid z = x2 y2 inside the cylinder x2 y2 = 4 oriented upward, and f(x,y,z) = x2z2i y2z2j xyzk. rather than evaluating rr s curlf ds, we simply compute. Stokes' theorem says that ∮c ⇀ f ⋅ d ⇀ r = ∬s ⇀ ∇ × ⇀ f ⋅ ˆn ds for any (suitably oriented) surface whose boundary is c. so if s1 and s2 are two different (suitably oriented) surfaces having the same boundary curve c, then. ∬s1 ⇀ ∇ × ⇀ f ⋅ ˆn ds = ∬s2 ⇀ ∇ × ⇀ f ⋅ ˆn ds. for example, if c is the unit.

Si 9 Example Of Stokes Theorem Circulation From The Flux Of The Curl

Comments are closed.